The cosine x equation is equal to a. Trigonometric equations - formulas, solutions, examples. Factorization

You can order a detailed solution to your problem !!!

An equality containing an unknown under the sign of a trigonometric function (`sin x, cos x, tan x` or` ctg x`) is called a trigonometric equation, and we will consider their formulas further.

The simplest equations are called `sin x = a, cos x = a, tg x = a, ctg x = a`, where` x` is the angle to be found, `a` is any number. Let's write down the root formulas for each of them.

1. Equation `sin x = a`.

For `| a |> 1` has no solutions.

For `| a | \ leq 1` has an infinite number of solutions.

Root formula: `x = (- 1) ^ n arcsin a + \ pi n, n \ in Z`

2. The equation `cos x = a`

For `| a |> 1` - as in the case of sine, it has no solutions among real numbers.

For `| a | \ leq 1` has an infinite number of solutions.

Root formula: `x = \ pm arccos a + 2 \ pi n, n \ in Z`

Special cases for sine and cosine in graphs.

3. The equation `tg x = a`

Has an infinite number of solutions for any values ​​of `a`.

Root formula: `x = arctan a + \ pi n, n \ in Z`

4. Equation `ctg x = a`

Also has an infinite number of solutions for any values ​​of `a`.

Root formula: `x = arcctg a + \ pi n, n \ in Z`

Formulas for roots of trigonometric equations in a table

For sine:
For cosine:
For tangent and cotangent:
Formulas for solving equations containing inverse trigonometric functions:

Methods for solving trigonometric equations

The solution to any trigonometric equation consists of two stages:

• using convert it to the simplest;
• solve the resulting simplest equation using the above written root formulas and tables.

Let's look at the examples of the main methods of solving.

Algebraic method.

In this method, variable replacement and substitution into equality is done.

Example. Solve the equation: `2cos ^ 2 (x + \ frac \ pi 6) -3sin (\ frac \ pi 3 - x) + 1 = 0`

`2cos ^ 2 (x + \ frac \ pi 6) -3cos (x + \ frac \ pi 6) + 1 = 0`,

we make the change: `cos (x + \ frac \ pi 6) = y`, then` 2y ^ 2-3y + 1 = 0`,

we find the roots: `y_1 = 1, y_2 = 1 / 2`, whence two cases follow:

1.` cos (x + \ frac \ pi 6) = 1`, `x + \ frac \ pi 6 = 2 \ pi n`,` x_1 = - \ frac \ pi 6 + 2 \ pi n`.

2.` cos (x + \ frac \ pi 6) = 1 / 2`, `x + \ frac \ pi 6 = \ pm arccos 1/2 + 2 \ pi n`,` x_2 = \ pm \ frac \ pi 3- \ frac \ pi 6 + 2 \ pi n`.

Answer: `x_1 = - \ frac \ pi 6 + 2 \ pi n`,` x_2 = \ pm \ frac \ pi 3- \ frac \ pi 6 + 2 \ pi n`.

Factorization.

Example. Solve the equation: `sin x + cos x = 1`.

Solution. Move all the terms of the equality to the left: `sin x + cos x-1 = 0`. Using, transform and factor the left side:

`sin x - 2sin ^ 2 x / 2 = 0`,

`2sin x / 2 cos x / 2-2sin ^ 2 x / 2 = 0`,

`2sin x / 2 (cos x / 2-sin x / 2) = 0`,

1. `sin x / 2 = 0`,` x / 2 = \ pi n`, `x_1 = 2 \ pi n`.
2. `cos x / 2-sin x / 2 = 0`,` tg x / 2 = 1`, `x / 2 = arctan 1+ \ pi n`,` x / 2 = \ pi / 4 + \ pi n` , `x_2 = \ pi / 2 + 2 \ pi n`.

Answer: `x_1 = 2 \ pi n`,` x_2 = \ pi / 2 + 2 \ pi n`.

Reduction to a homogeneous equation

First, you need to bring this trigonometric equation to one of two types:

`a sin x + b cos x = 0` (homogeneous equation of the first degree) or` a sin ^ 2 x + b sin x cos x + c cos ^ 2 x = 0` (homogeneous equation of the second degree).

Then divide both parts by `cos x \ ne 0` - for the first case, and by` cos ^ 2 x \ ne 0` - for the second. We obtain equations for `tg x`:` a tg x + b = 0` and `a tg ^ 2 x + b tg x + c = 0`, which need to be solved by known methods.

Example. Solve the equation: `2 sin ^ 2 x + sin x cos x - cos ^ 2 x = 1`.

Solution. Rewrite the right side as `1 = sin ^ 2 x + cos ^ 2 x`:

`2 sin ^ 2 x + sin x cos x - cos ^ 2 x =` `sin ^ 2 x + cos ^ 2 x`,

`2 sin ^ 2 x + sin x cos x - cos ^ 2 x -` `sin ^ 2 x - cos ^ 2 x = 0`

`sin ^ 2 x + sin x cos x - 2 cos ^ 2 x = 0`.

This is a homogeneous trigonometric equation of the second degree, we divide its left and right sides by `cos ^ 2 x \ ne 0`, we get:

`\ frac (sin ^ 2 x) (cos ^ 2 x) + \ frac (sin x cos x) (cos ^ 2 x) - \ frac (2 cos ^ 2 x) (cos ^ 2 x) = 0`

`tg ^ 2 x + tg x - 2 = 0`. We introduce the replacement `tg x = t`, as a result,` t ^ 2 + t - 2 = 0`. The roots of this equation are `t_1 = -2` and` t_2 = 1`. Then:

1. `tg x = -2`,` x_1 = arctg (-2) + \ pi n`, `n \ in Z`
2. `tg x = 1`,` x = arctan 1+ \ pi n`, `x_2 = \ pi / 4 + \ pi n`,` n \ in Z`.

Answer. `x_1 = arctg (-2) + \ pi n`,` n \ in Z`, `x_2 = \ pi / 4 + \ pi n`,` n \ in Z`.

Go to half corner

Example. Solve the equation: `11 sin x - 2 cos x = 10`.

Solution. Apply the double angle formulas, as a result: `22 sin (x / 2) cos (x / 2) -`` 2 cos ^ 2 x / 2 + 2 sin ^ 2 x / 2 =` `10 sin ^ 2 x / 2 +10 cos ^ 2 x / 2`

`4 tg ^ 2 x / 2 - 11 tg x / 2 + 6 = 0`

Applying the above algebraic method, we get:

1. `tg x / 2 = 2`,` x_1 = 2 arctan 2 + 2 \ pi n`, `n \ in Z`,
2. `tg x / 2 = 3 / 4`,` x_2 = arctan 3/4 + 2 \ pi n`, `n \ in Z`.

Answer. `x_1 = 2 arctan 2 + 2 \ pi n, n \ in Z`,` x_2 = arctan 3/4 + 2 \ pi n`, `n \ in Z`.

Introduce an auxiliary corner

In the trigonometric equation `a sin x + b cos x = c`, where a, b, c are coefficients and x is a variable, we divide both sides by` sqrt (a ^ 2 + b ^ 2) `:

`\ frac a (sqrt (a ^ 2 + b ^ 2)) sin x +` `\ frac b (sqrt (a ^ 2 + b ^ 2)) cos x = '' \ frac c (sqrt (a ^ 2 + b ^ 2)) `.

The coefficients on the left side have the properties of sine and cosine, namely, the sum of their squares is equal to 1 and their absolute values ​​are not greater than 1. We denote them as follows: `\ frac a (sqrt (a ^ 2 + b ^ 2)) = cos \ varphi` , `\ frac b (sqrt (a ^ 2 + b ^ 2)) = sin \ varphi`,` \ frac c (sqrt (a ^ 2 + b ^ 2)) = C`, then:

`cos \ varphi sin x + sin \ varphi cos x = C`.

Let's take a closer look at the following example:

Example. Solve the equation: `3 sin x + 4 cos x = 2`.

Solution. Divide both sides of the equality by `sqrt (3 ^ 2 + 4 ^ 2)`, we get:

`\ frac (3 sin x) (sqrt (3 ^ 2 + 4 ^ 2)) +` `\ frac (4 cos x) (sqrt (3 ^ 2 + 4 ^ 2)) = '' \ frac 2 (sqrt (3 ^ 2 + 4 ^ 2)) `

`3/5 sin x + 4/5 cos x = 2 / 5`.

Let's denote `3/5 = cos \ varphi`,` 4/5 = sin \ varphi`. Since `sin \ varphi> 0`,` cos \ varphi> 0`, then we take `\ varphi = arcsin 4 / 5` as an auxiliary angle. Then we write our equality in the form:

`cos \ varphi sin x + sin \ varphi cos x = 2 / 5`

Applying the formula for the sum of the angles for the sine, we write our equality in the following form:

`sin (x + \ varphi) = 2 / 5`,

`x + \ varphi = (- 1) ^ n arcsin 2/5 + \ pi n`,` n \ in Z`,

`x = (- 1) ^ n arcsin 2/5-` `arcsin 4/5 + \ pi n`,` n \ in Z`.

Answer. `x = (- 1) ^ n arcsin 2/5-` `arcsin 4/5 + \ pi n`,` n \ in Z`.

Fractional-rational trigonometric equations

These are equalities with fractions with trigonometric functions in the numerators and denominators.

Example. Solve the equation. `\ frac (sin x) (1 + cos x) = 1-cos x`.

Solution. Multiply and divide the right side of the equality by `(1 + cos x)`. As a result, we get:

`\ frac (sin x) (1 + cos x) = '' \ frac ((1-cos x) (1 + cos x)) (1 + cos x)`

`\ frac (sin x) (1 + cos x) =` `\ frac (1-cos ^ 2 x) (1 + cos x)`

`\ frac (sin x) (1 + cos x) =` `\ frac (sin ^ 2 x) (1 + cos x)`

`\ frac (sin x) (1 + cos x) -`` \ frac (sin ^ 2 x) (1 + cos x) = 0`

`\ frac (sin x-sin ^ 2 x) (1 + cos x) = 0`

Considering that the denominator cannot be equal to zero, we get `1 + cos x \ ne 0`,` cos x \ ne -1`, `x \ ne \ pi + 2 \ pi n, n \ in Z`.

Equate the numerator of the fraction to zero: `sin x-sin ^ 2 x = 0`,` sin x (1-sin x) = 0`. Then `sin x = 0` or` 1-sin x = 0`.

1. `sin x = 0`,` x = \ pi n`, `n \ in Z`
2. `1-sin x = 0`,` sin x = -1`, `x = \ pi / 2 + 2 \ pi n, n \ in Z`.

Considering that `x \ ne \ pi + 2 \ pi n, n \ in Z`, the solutions are` x = 2 \ pi n, n \ in Z` and `x = \ pi / 2 + 2 \ pi n` , `n \ in Z`.

Answer. `x = 2 \ pi n`,` n \ in Z`, `x = \ pi / 2 + 2 \ pi n`,` n \ in Z`.

Trigonometry, and trigonometric equations in particular, are used in almost all areas of geometry, physics, engineering. The study begins in grade 10, there are definitely tasks for the exam, so try to remember all the formulas of trigonometric equations - they will definitely come in handy!

However, you don't even need to memorize them, the main thing is to understand the essence and be able to deduce them. It's not as difficult as it sounds. See for yourself by watching the video.

The simplest trigonometric equations are usually solved by formulas. Let me remind you that the following trigonometric equations are called the simplest:

sinx = a

cosx = a

tgx = a

ctgx = a

x is the angle to be found,
a - any number.

And here are the formulas with which you can immediately write down the solutions of these simplest equations.

For sine:

For cosine:

х = ± arccos a + 2π n, n ∈ Z

For tangent:

x = arctan a + π n, n ∈ Z

For cotangent:

x = arcctg a + π n, n ∈ Z

Actually, this is the theoretical part of solving the simplest trigonometric equations. Moreover, everything!) Nothing at all. However, the number of errors on this topic is simply off scale. Especially if the example deviates slightly from the template. Why?

Yes, because a lot of people write down these letters, not understanding their meaning at all! With caution he writes down, no matter how something happens ...) This must be dealt with. Trigonometry for humans, or humans for trigonometry after all !?)

Shall we figure it out?

One angle will be equal to arccos a, second: -arccos a.

And it will always work that way. For any a.

If you don't believe me, hover your mouse over the picture, or tap the picture on the tablet.) I changed the number a to some negative. Anyway, we got one corner arccos a, second: -arccos a.

Therefore, the answer can always be written in the form of two series of roots:

x 1 = arccos a + 2π n, n ∈ Z

x 2 = - arccos a + 2π n, n ∈ Z

We combine these two series into one:

x = ± arccos a + 2π n, n ∈ Z

And that's all. Got a general formula for solving the simplest trigonometric equation with cosine.

If you understand that this is not some kind of super-scientific wisdom, but just an abbreviated notation of two series of responses, you and the task "C" will be on the shoulder. With inequalities, with the selection of roots from a given interval ... There the answer with plus / minus does not roll. And if you treat the answer in a businesslike manner, and break it down into two separate answers, everything is decided.) Actually, for this we understand. What, how and where.

In the simplest trigonometric equation

sinx = a

also two series of roots are obtained. Is always. And these two series can also be recorded one line. Only this line will be more cunning:

х = (-1) n arcsin a + π n, n ∈ Z

But the essence remains the same. Mathematicians simply constructed a formula to make one instead of two records of a series of roots. And that's it!

Let's check the mathematicians? And then you never know ...)

In the previous lesson, the solution (without any formulas) of a trigonometric equation with a sine was analyzed in detail:

The answer produced two series of roots:

x 1 = π / 6 + 2π n, n ∈ Z

x 2 = 5π / 6 + 2π n, n ∈ Z

If we solve the same equation using the formula, we get the answer:

x = (-1) n arcsin 0.5 + π n, n ∈ Z

Actually, this is an unfinished answer.) The student must know that arcsin 0.5 = π / 6. A complete answer would be:

x = (-1) n π / 6+ π n, n ∈ Z

This raises an interesting question. Reply via x 1; x 2 (that's the right answer!) and through the lonely NS (and this is the correct answer!) - the same thing, or not? We'll find out now.)

Substitute in response with x 1 meaning n = 0; 1; 2; and so on, we count, we get a series of roots:

x 1 = π / 6; 13π / 6; 25π / 6 etc.

With the same substitution in the answer with x 2 , we get:

x 2 = 5π / 6; 17π / 6; 29π / 6 etc.

Now we substitute the values n (0; 1; 2; 3; 4 ...) into the general formula for a lonely NS ... That is, we raise minus one to zero, then to the first, second, etc. And, of course, we substitute 0 in the second term; 1; 2 3; 4, etc. And we count. We get the series:

x = π / 6; 5π / 6; 13π / 6; 17π / 6; 25π / 6 etc.

That's all you can see.) The general formula gives us exactly the same results, as the two answers separately. Only all at once, in order. Don't be fooled by the mathematicians.)

Formulas for solving trigonometric equations with tangent and cotangent can also be checked. But we will not.) They are so simple.

I have described all this substitution and verification on purpose. It is important to understand one simple thing here: there are formulas for solving elementary trigonometric equations, just a short record of the answers. For this brevity, I had to insert plus / minus in the cosine solution and (-1) n in the sine solution.

These inserts do not interfere in any way in tasks where you just need to write down the answer to an elementary equation. But if you need to solve inequality, or then you need to do something with the answer: select roots on an interval, check for ODZ, etc., these inserts can easily unsettle a person.

And what to do? Yes, either write down the answer in two series, or solve the equation / inequality along the trigonometric circle. Then these inserts disappear and life becomes easier.)

We can summarize.

There are ready-made answer formulas for solving the simplest trigonometric equations. Four pieces. They are good for instantly recording the solution to an equation. For example, you need to solve the equations:

sinx = 0.3

Easily: х = (-1) n arcsin 0,3 + π n, n ∈ Z

cosx = 0.2

No problem: х = ± arccos 0,2 + 2π n, n ∈ Z

tgx = 1.2

Easily: x = arctan 1,2 + π n, n ∈ Z

ctgx = 3.7

One left: x = arcctg3,7 + π n, n ∈ Z

cos x = 1.8

If you, shining with knowledge, instantly write the answer:

x = ± arccos 1,8 + 2π n, n ∈ Z

then you already shine, this ... that ... from the puddle.) The correct answer: no solutions. Do you understand why? Read what the arccosine is. In addition, if the tabular values ​​of sine, cosine, tangent, cotangent are on the right side of the original equation, - 1; 0; √3; 1/2; √3/2 etc. - the answer through the arches will be unfinished. Arches must be translated into radians.

And if you come across inequality like

then the answer is:

х πn, n ∈ Z

there is a rare nonsense, yes ...) Here it is necessary to decide on the trigonometric circle. What we will do in the relevant topic.

For those who have heroically read up to these lines. I just can't help but appreciate your titanic efforts. You a bonus.)

Bonus:

When writing formulas in an alarming combat environment, even academically hardened nerds often get confused about where πn, And where 2π n. Here's a simple trick. In of all formulas worth πn. Except for the only formula with inverse cosine. It stands there 2πn. Two pien. Keyword - two. The same formula contains two sign at the beginning. Plus and minus. Here and there - two.

So if you wrote two sign in front of the inverse cosine, it is easier to remember what will be at the end two pien. And even the opposite happens. Skip man sign ± , gets to the end, writes it right two pien, and it will come to its senses. Ahead of something two sign! The person will return to the beginning, but he will correct the mistake! Like this.)

If you like this site ...

By the way, I have a couple more interesting sites for you.)

You can practice solving examples and find out your level. Instant validation testing. Learning - with interest!)

you can get acquainted with functions and derivatives.

Zakharova Ludmila Vladimirovna
MBOU "Secondary School No. 59" in Barnaul
mathematic teacher [email protected]

№1 Simplest trigonometric equations

Target: 1. Derive formulas for solutions of the simplest trigonometric equations of the form sinx = a, cosx = a, tgx = a, ctgx = a;

2. Learn to solve the simplest trigonometric equations using formulas.

Equipment: 1) Tables with graphs of trigonometric functions y = sinx, y = cosx, y = tgx, y = ctgx; 2) Table of values ​​of inverse trigonometric functions; 3) Summary table of formulas for solving the simplest trigonometric equations.

Lecture lesson plan:

1 . Derivation of formulas for the roots of the equation

a) sinx = a,

b) cosx = a,

c) tgx = a,

d) ctgx = a.

2 ... Oral frontal work to consolidate the formulas obtained.

3 ... Written work to consolidate the studied material

In algebra, geometry, physics and other subjects, we are faced with a variety of problems, the solution of which is associated with the solution of equations. We have studied the properties of trigonometric functions, so it is natural to turn to equations in which the unknown is contained under the sign of the functions

Definition: Equations of the form sinx = a , cosx= a , tgx= a , ctgx= a are called the simplest trigonometric equations.

It is very important to learn how to solve the simplest trigonometric equations, since all methods and techniques for solving any trigonometric equations are to reduce them to the simplest ones.

Let's start by deriving formulas that "actively" work when solving trigonometric equations.

1.Equations of the form sinx = a.

Let's solve the equation sinx = a graphically. To do this, in one coordinate system, we plot the graphs of the functions y = sinx and y = a.

1) If a> 1 and a sin x = a has no solutions, since the straight line and the sinusoid have no points in common.

2) If -1a a crosses the sinusoid infinitely many times. This means that the equation sinx = a has infinitely many solutions.

Since the period of the sine is 2 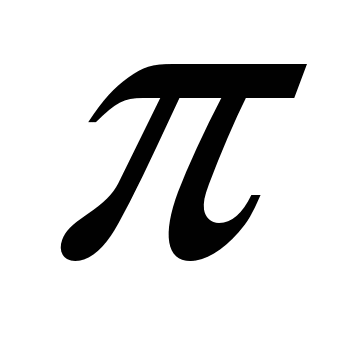, then to solve the equation sinx = a it is enough to find all solutions on any segment of length 2.

By solving the equation for [- / 2; / 2] by the definition of the arcsine x = arcsin a, and on x = -arcsin a... Taking into account the periodicity of the function y = sinx, we obtain the following expressions

x = -arcsin a+ 2n, n Z.

Both series of solutions can be combined

X = (-1) n arcsin a+ n, nZ.

In the following three cases, they prefer to use not the general formula, but simpler relations:

If a= -1, then sin x = -1, x = - / 2 + 2n

If a= 1, then sin x = 1, x = / 2 + 2n

If a = 0, then sin x = 0. x = n,

Example: Solve Equation sinx = 1/2.

Let's compose the formulas for the solutions x = arcsin 1/2 + 2n

X = - arcsin a + 2n

Let's calculate the value arcsin1 / 2. Substitute the found value into the solution formulas

x = 5/6 + 2 n

or by the general formula

X = (-1) n arcsin 1/2 + n,

X = (-1) n / 6 + n,

2. Equations of the form cosx = a.

Let's solve the equation cosx = a also graphically, plotting the graphs of the functions y = cosx and y = a.

1) If a 1, then the equation cosx = a has no solutions, since the graphs do not have common points.

2) If -1 a cosx = a has an infinite number of solutions.

We will find all the solutions cosx = a on an interval of length 2 since the period of the cosine is 2.

On the solution of the equation by definition of the arccosine will be x = arcos a. Given the parity of the cosine function, the solution to the equation on [-; 0] will be x = -arcos a.

Thus, the solutions to the equation cosx = a x = + arcos a+ 2 n,

In three cases, we will use not a general formula, but simpler relations:

If a= -1, then cosx = -1, x = - / 2 + 2n

If a= 1, then cosx = 1, x = 2n,

If a = 0, then cosx = 0. x = / 2 + n

Example: Solve Equation cos x = 1/2,

Let's compose the formulas for the solutions x = arccos 1/2 + 2n

Let's calculate the value arccos1 / 2.

Substitute the found value into the solution formulas

X = + /3+ 2n, nZ.

Equations of the form tgx = a.

Since the period of the tangent is equal, then in order to find all the solutions of the equation tgx = a, it is enough to find all solutions on any interval of length. By the definition of the arctangent, the solution to the equation on (- / 2; / 2) is arctan a. Taking into account the period of the function, all solutions of the equation can be written in the form

x = arctg a+ n, nZ.

Example: Solve the equation tg x = 3/3

Let's compose a formula for solving x = arctan 3/3 + n, nZ.

We calculate the value of the arctangent arctan 3/3 = / 6, then

X = / 6 + n, nZ.

Derivation of a formula for solving an equation with tgx= a can be provided to students.

Example.

Solve the equation ctg x = 1.

x = arcсtg 1 + n, nZ,

X = / 4 + n, nZ.

As a result of the material studied, students can fill out the table:

"Solving trigonometric equations."

the equation

Exercises to consolidate the material studied.

(Orally) Which of the written equations can be solved by the formulas:

a) x = (-1) n arcsin a+ n, nZ;

b) x = + arcos a + 2 n?

cos x = 2/2, tg x = 1, sin x = 1/3, ctg x = 3/3, sin x = -1/2, cos x = 2/3, sin x = 3, cos x = 2 ...

Which of the listed equations have no solutions?

Solve the equations:

a) sin x = 0; e) sin x = 2/2; h) sin x = 2;

b) cos x = 2/2; f) cos x = -1/2; i) cos x = 1;

d) tg x = 3; g) ctg x = -1; j) tg x = 1/3.

3. Solve the equations:

a) sin 3x = 0; e) 2cos x = 1;

b) cos x / 2 = 1/2; f) 3 tan 3x = 1;

d) sin x / 4 = 1; g) 2cos (2x + / 5) = 3.

When solving these equations, it is useful to write down the rules for solving equations of the form sin v x = a, and with sin v x = a, | a|1.

Sin v x = a, | a | 1.

v x = (-1) n arcsin a+ n, nZ,

x = (-1) n 1 / v arcsin a+ n / v, nZ.

Summing up the results of the lesson:

Today in the lesson we have derived formulas for solving the simplest trigonometric equations.

Dismantled examples of solving the simplest trigonometric equations.

We filled in the table that we will use to solve the equations.

Homework.

№2 Solving trigonometric equations

Target: To study methods for solving trigonometric equations: 1) reducible to quadratic; 2) reducible to homogeneous trigonometric equations.

To develop students' observation skills when using various methods of solving trigonometric equations.

Frontal work with students.

What are the formulas for the roots of trigonometric equations cos x = a, sin x = a, tgx = a, ctg x = a.

Solve the equations (orally):

cos x = -1, sin x = 0, tgx = 0, ctg x = 1, cos x = 1.5, sin x = 0.

Find errors and think about the causes of errors.

cos x = 1/2, x = + / 6 + 2k, k Z.

sin x = 3/2, x = / 3 + k, kZ.

tgx = / 4, x = 1 + k, kZ.

2. Learning new material.

This lesson will cover some of the most common methods for solving trigonometric equations.

Trigonometric equations reduced to quadratic.

This class can include equations that include one function (sine or cosine) or two functions of one argument, but one of them is reduced to the second with the help of basic trigonometric identities.

For example, if cоsх is included in the equation in even powers, then we replace it with 1- sin 2 x, if sin 2 x, then we replace it with 1-cos 2 x.

Example.

Solve the equation: 8 sin 2 x - 6sin x -5 = 0.

Solution: We denote sin x = t, then 8t 2 - 6t - 5 = 0,

D = 196,

T 1 = -1/2, t 2 = -5/4.

Let's perform the reverse change and solve the following equations.

X = (- 1) k + 1/6 + k, kZ.

Since -5/4> 1, the equation has no roots.

Answer: x = (- 1) k + 1/6 + k, kZ.

Solution of strengthening exercises.

Solve the equation:

1) 2sin 2 x + 3cos x = 0;

2) 5sin 2 x + 6cos x -6 = 0;

3) 2sin 2 x + 3cos 2 x = -2sin x;

4) 3 tg 2 x +2 tgx-1 = 0.

Homogeneous trigonometric equations.

Definition: 1) Equation of the forma sinx + b cosx= 0, (a = 0, b = 0) is called a homogeneous equation of the first degree with respect to sin x and cos x.

This equation is solved by dividing both parts of it by cosx 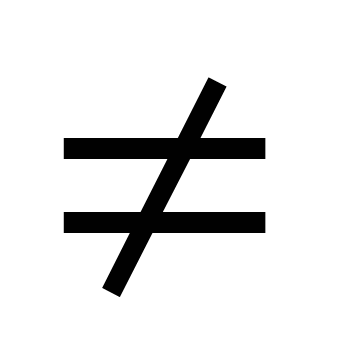0. The result is the equation atgx + b = 0.

2) An equation of the forma sin 2 x + b sinx cosx + c cos 2 x =0 is called a homogeneous equation of the second degree, where a, b, c are any numbers.

If a = 0, then the equation is solved by dividing both parts by cos 2 x 0. As a result, we obtain the equation atg 2 x + btgx + c = 0.

Comment: Equation of the forma sin mx + b cos mx=0 or

a sin 2 mx + b sin mx cos mx + c cos 2 mx =0 are also homogeneous. To solve them, both sides of the equation are divided by cos mx=0 or cos 2 mx=0

3) Various equations can be reduced to homogeneous equations, which initially are not such. For example,sin 2 mx + b sin mx cos mx + c cos 2 mx = d, and a sinx + b cosx= d. To solve these equations, you need to multiply the right side by "Trigonometric unit" those. on sin 2 x + cos 2 x and perform mathematical transformations.

Exercises to consolidate the studied material:

1) 2sin x- 3cos x = 0; 5) 4 sin 2 x - sin2x = 3;

2) sin 2x + cos2x = 0; 6) 3 sin 2 x + sinx cosx = 2 cos 2 x;

3) sin x + 3cos x = 0; 7) 3 sin 2 x- sinx cosx = 2;

4) sin 2 x -3 sinx cosx +2 cos 2 x = 0

3. Summing up the results of the lesson. Homework.

In this lesson, depending on the preparedness of the group, you can consider solving equations of the form a sin mx + b cos mx = c, where a, b, c are not equal to zero at the same time.

Strengthening exercises:

1.3sin x + cos x = 2;

2.3sin 2x + cos 2x = 2;

3.sin x / 3 + cos x / 3 = 1;

4.12 sin x +5 cos x + 13 = 0.

№ 3 Solving trigonometric equations

Target: 1) Study the method of solving trigonometric equations by factorization; learn to solve trigonometric equations using various trigonometric formulas;

2) Check: students' knowledge of formulas for solving the simplest trigonometric equations; the ability to solve the simplest trigonometric equations.

Lesson plan:

Homework check.

Mathematical dictation.

Learning new material.

Independent work.

Summing up the results of the lesson. Homework.

Course of the lesson:

Homework check (the solution to the trigonometric equations is briefly written on the blackboard).

Mathematical dictation.

IN 1

1. What equations are called the simplest trigonometric equations?

2. What is the name of an equation of the forma sinx + b cosx = 0? Please indicate the way to solve it.

3.Write the formula for the roots of the equation tgx = a(ctg x = a).

4. Write down the formulas for the roots of equations of the form cosx = a, where a=1, a=0, a=-1.

5. Write down the general formula for the roots of the equation sin x = a, | a|

6. How are equations of the forma cosx = b, | b|

IN 2

1. Write down the formulas for the roots of the equations cosx = a,| a|

2. Write down the general formula for the roots of the equation

= a, | a|

3. What are the names of equations of the form sin x = a, tgx = a, sin x = a?

4. Write down the formulas for the roots of the equation sin x = a, if a=1, a=0, a=-1.

5.How are equations of the form sin a x = b, | b|

6. What equations are called homogeneous equations of the second degree? How are they resolved?

Learning new material.

Factoring method.

One of the most commonly used methods for solving trigonometric equations is the factorization method.

If the equation f (x) = 0 can be represented as f 1 (x) f 2 (x) = 0, then the problem is reduced to solving two equations f 1 (x) = 0, f 2 (x) = 0.

(With students, it is helpful to remember the rule “ The product of the factors is zero if at least one of the factors is zero, while the others make sense»)

Consolidation of the studied material through the solution of equations of varying complexity.

(sin x-1/2) (sin x + 1) = 0; 2) (cosx- 2/2) (sin x + 2/2) = 0; (self)

3) sin 2 x + sin x cosx = 0; 4) sin 2 x - sin x = 0;

5) sin 2x - cosx = 0; 6) 4 cos 2 x -1 = 0; (In 2 ways)

7) cosx + cos3x = 0; 8) sin 3x = sin 17x;

9) sin x + sin 2x + sin 3x = 0; 10) cos3x cos5x

11) sin x cos5x = sin 9x cos3x sin 2x sin 2x

12) 3 cosx sin x + cos 2 x = 0 (self)

13) 2 cos 2 x - sin (x- / 2) + tgx tg (x + / 2) = 0.

Independent work.

Option-1 Option-2

1) 6 sin 2 x + 5sin x -1 = 0; 1) 3 cos 2 x + 2 cosx -5 = 0;

2) sin 2x - cos2x = 0; 2) 3 cos x / 2 - sin x / 2 = 0;

3) 5 sin 2 x + sin x cosx -2 cos 2 x = 2; 3) 4sin 2 x- sin x cosx + 7cos 2 x = 5;

4) sin x + sin5x = sin3x + sin7x; 4) sin x-sin 2x + sin 3x-sin 4x = 0;

5) sin x + cosx = 1. 5) sin x + cosx = 2.

8. Summing up the lesson. Homework.